Trip on the Complex Plane

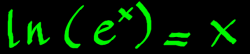
Logarithms

This page will lead to the plane of complex logarithms. That plane is again drawn on the usual squared paper. But familiar references of the normal complex plane, like the origin, axes and unit circle, will be radically transformed. The spiral plane with it's stack of branches like we have seen it on the previous page, will be flattened out, thereby stretching the unit circle to a straight line and projecting the origin to... ah we'll see that. Once you have a complex exponential, finding the logarithm of it is as easy as can be.

Taking logarithms is the inverse process of exponenting. So, for real exponentials we have:

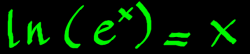Where ln means natural logarithm, the base e logarithm. For complex exponentials and logarithms, the equivalent is:

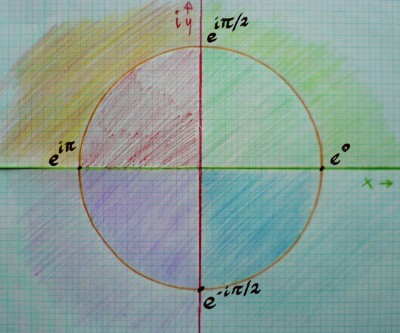
Below are two examples of complex exponentials on the unit circle and natural logarithms of these. The logarithms are on the iy-axis because the exponentials had pure imaginary exponents. This holds for all exponentials on the unit circle. So their logs will all go to the iy-axis.

The circle will unroll to a flat vertical line. And what will happen to the axes that crossed the circle? We will try to do some visuals on the unrolling.

Take the thing apart at the position of the branch cut, and stretch.

Looks like a bunch of mikado sticks. This stage has no simple arithmetical analogy I guess.

The former unit circle now becomes the iy-axis. The former vertical axis is split, and lying horizontally below and above the new real axis. And what is that green line in the back there? The former negative x-axis went there. Maybe that part of the real axis was not so real after all.

And what about the origin of the complex plane, where did it go?

It will never be seen here, on the plane of complex logarithms. Ask your calculator what is the log of zero. It will answer: math error, exception, or at best minus infinity. And that is where the original origin went. It is spread past the horizon, at all minus infinities.

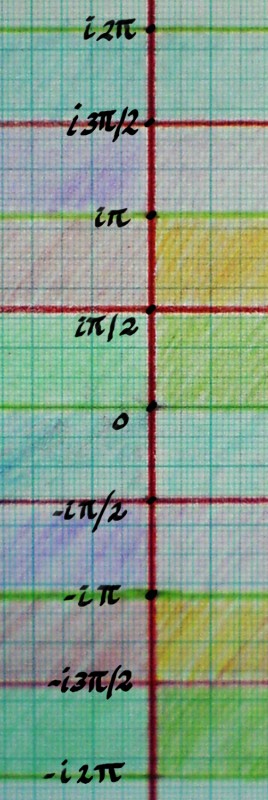
Below, the transformation is illustrated again, but now with teinted quadrants, to track where they go.

Now recall that the complex plane has branches. On the plane of complex logarithms, these branches are no longer piled up to overlap one another. Instead, they appear neatly above and below their neighbours on one and the same plane. The horizontal green and red lines are the logarithms of the recurrent x and iy axes of the complex plane branches. Here, i*2pi is at a different location than i*200*pi for example. But do not be fooled. Once you revert to the normal complex plane by transforming the logarithms into their exponential form, the overlap is there again. Therefore, complex logarithms are not so unique as they appear here. Then what is the use of complex logarithms if they are not more unique than the exponentials? Let us not sink too deep into mathematical theory, but go back to amplitudes and frequencies. Because they are the reason for using the logarithms.

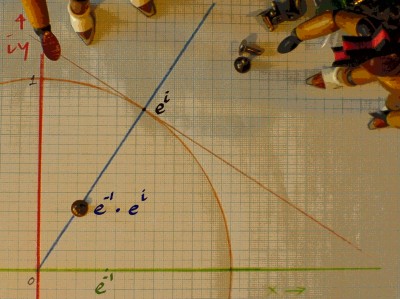
On one of the previous pages we plotted the point e^(-1+i), which is e^-1*eⁱ. We found that e^-1 is the radius, while eⁱ sets the arc length of one radian on the unit circle. The logarithm of it, (-1+i), is now plotted for comparison.

On the plane of complex logarithms, rotation in radians is expressed with a number on the iy-axis. It contains more information than just an arc length because it has a direction too, inherited from the complex exponential that had a positive or negative imaginary power.

Here we finally have a number directly expressing rotation or (when related to time) frequency. With directly I mean: being a number and not an exponent of something. Because, e^ix is not an angle but ln(e^ix) is. Let us be happy with this result for a moment, before we turn to a more enigmatic side of the complex logarithms.

On the x-axis of the plane of complex logarithms is the logarithm of the radius. So, obviously, not the radius itself. I find these things quite intriguing. Apperently, an angle is a logarithm of something, and a radius is an exponential of something. What does it mean: the logarithm of a radius length? I think it means this:

The logarithm of the radius is a real number, expressing a distance from unity radius, or unit amplitude. The complement of that distance is not a solid length, like a radius seems to have, but the indefinite remainder between minus infinity and that number.

Weird as it may seem, this perspective is not unrealistic at all. The unit circle on the complex plane is the actual reference not only for angles, but also for radiuses. A radius is best considered as a ratio of unit length. Or as an exponential of some rise or decay number, which has unity amplitude as it's reference. The 'origin' at the centre of the unit circle, is not the point where things originate, but rather a point where things vanish. A black hole...

The plane of complex logarithms may be the point of departure when doing sound synthesis or filters, and a point of arrival when doing frequency analysis. Because it is here that angles and thus frequencies can be expressed. Via complex exponentials they are connected to complex vectors of the 'normal' complex plane. And via these to the world of real sample values.